I asserted that this forum could do with more 101-level and/or mathematical and/or falsifiable posts, and people agreed with me, so here is one. People confident in highschool math mostly won’t get much out of most of this, but students browsing this site between lectures might.
The Sine Rule
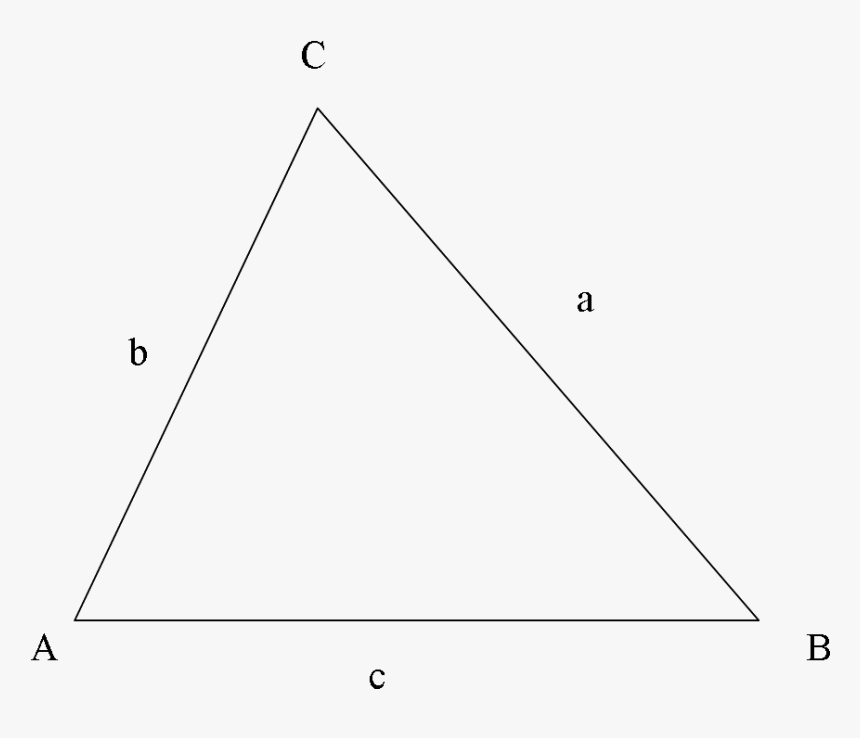
Say you have a triangle with side lengths a, b, c and internal angles A, B, C. You know a, know A, know b, and want to know B. You could apply the Sine Rule. Or you could apply common sense: “A triangle has the same area as itself”. [1]
The area of a triangle is half the base times the height. If you treat a as the base, the height is c*sin(B). So the area is a*c*sin(B)/2. But if you treat b as the base, the height is c*sin(A). So the area is also b*c*sin(A)/2. So a*c*sin(B)/2 = b*c*sin(A)/2. [...]
---
Outline:
(00:22) The Sine Rule
(02:01) Bayes' Theorem
(04:43) Integration By Parts
(07:03) Conclusion
The original text contained 4 footnotes which were omitted from this narration.
---
First published:
March 19th, 2025
Source:
https://www.lesswrong.com/posts/FR2aPsEcaRfy7iKZW/equations-mean-things
Narrated by TYPE III AUDIO.
---
Images from the article:
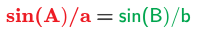
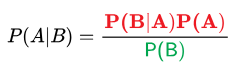



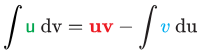


Apple Podcasts and Spotify do not show images in the episode description. Try Pocket Casts, or another podcast app.